Publications
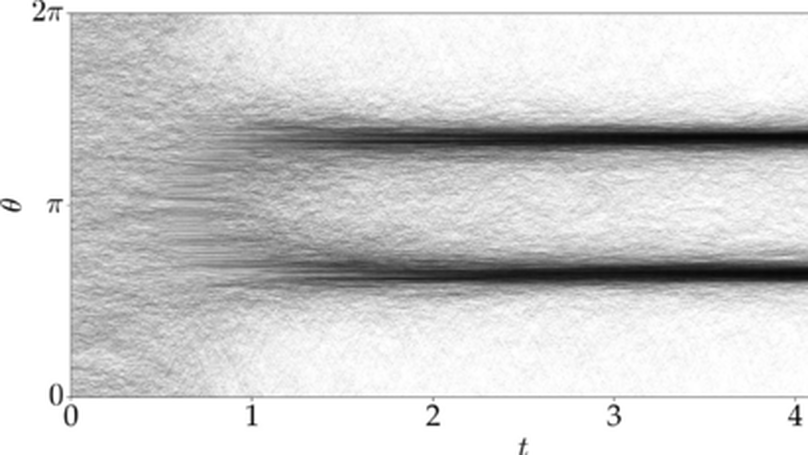
Collective synchronization through noise cancellation
After decades of study, there are only two known mechanisms to induce global synchronization in a population of oscillators: deterministic coupling and common forcing. The inclusion of independent noise in these models typically serves to drive disorder, increasing the stability of the incoherent state. Here we show that the reverse is also possible. We propose and analyze a simple general model of purely noise coupled oscillators. In the first explicit choice of noise coupling, we find the linear response around incoherence is identical to that of the paradigmatic Kuramoto model but exhibits binary phase locking instead of full coherence. We characterize the phase diagram, stationary states, and approximate low-dimensional dynamics for the model, revealing the curious behavior of this mechanism of synchronization. In the second minimal case we connect the final synchronized state to the initial conditions of the system.
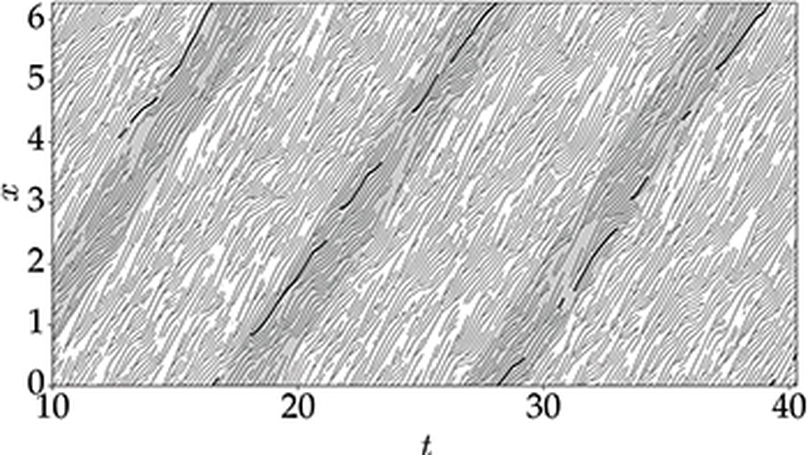
Stay in your lane: Density fluctuations in multi-lane traffic
When a new vehicle joins a lane, those behind may have to temporarily slow to accommodate them. Changing lane can be forced due to lane drops or junctions, but may also take place spontaneously at discretion of drivers, and recent studies have found that traffic jams and traffic oscillations can form even without such bottlenecks. Understanding how lane changing behaviour affects traffic flow is important for learning how to design roads and control traffic more effectively. Here, we present a stochastic model of spontaneous lane changing which exhibits a reduction in the overall flow of traffic. By examining the average flow rate both analytically and through simulations we find a definitive slow down of vehicles due to random switching between lanes. This results in the fundamental diagram depending on the rate of lane switching. By extending the model to three-lane traffic we find a larger impact on the flow of the middle lane compared to the side lanes.
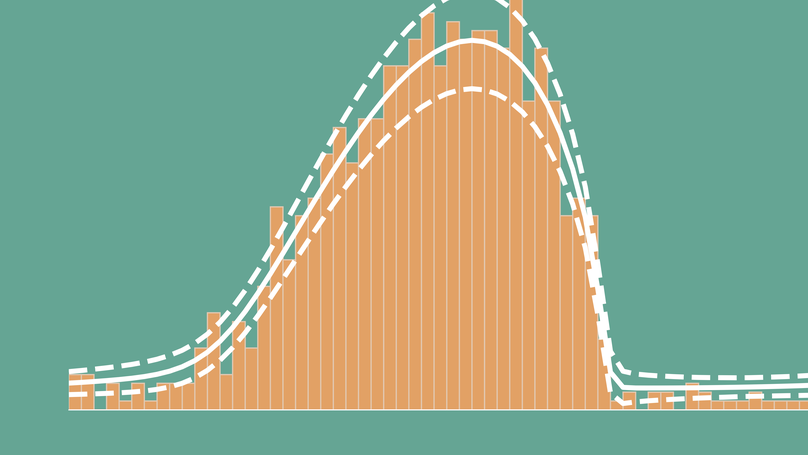
Density Fluctuations in Stochastic Kinematic Flows
At the macroscopic scale, many important models of collective motion fall into the class of kinematic flows for which both velocity and diffusion terms depend only on particle density. When total particle numbers are fixed and finite, simulations of corresponding microscopic dynamics exhibit stochastic effects which can induce a variety of interesting behaviours not present in the large system limit. In this article we undertake a systematic examination of finite-size fluctuations in a general class of particle models whose statistics correspond to those of stochastic kinematic flows. Doing so, we are able to characterise phenomena including: quasi-jams in models of traffic flow; stochastic pattern formation amongst spatially-coupled oscillators; anomalous bulk sub-diffusion in porous media; and travelling wave fluctuations in a model of bacterial swarming.